nawerassist.blogg.se

#Derivative of log base 2 x how to

Since the base of a logarithm can never be 1, then the only solution is 3. Rewrite the logarithm in exponential form to get Using the product rule Log b (m n) = log b m + log b n we get Write the logarithm in exponential form as įind the square root of both sides of the equation to get īut since, the base of logarithms can never be negative or 1, therefore, the correct answer is 30. Now, solve for x in the algebraic equation. Rewrite the logarithm in exponential form as Solve for x in the following logarithmic function log 2 (x – 1) = 5.

Rewrite the logarithmic function log 2( x) = 4 to exponential form. If 2 log x = 4 log 3, then find the value of ‘x’.įind the logarithm of 1024 to the base 2. Write the logarithmic equivalent of 5 3 = 125. Therefore, 7 2 = 64 in logarithmic function is Here, the base = 7, exponent = 2 and the argument = 49. Rewrite exponential function 7 2 = 49 to its equivalent logarithmic function. Let’s use these properties to solve a couple of problems involving logarithmic functions.

#Derivative of log base 2 x how to

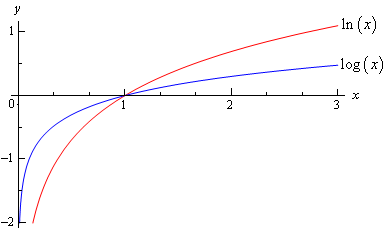
The third column tells about how to read both the logarithmic functions. The following table tells the way of writing and interchanging the exponential functions and logarithmic functions. Both of these functions are interchangeable. For that, you use an exponential function. Whenever you see logarithms in the equation, you always think of how to undo the logarithm to solve the equation. Always assume a base of 10 when solving with logarithmic functions without a small subscript for the base.Ĭomparison of exponential function and logarithmic function A logarithmic function with base 10is called a common logarithm.The base of logarithms can never be negative or 1.

$derivative of log base 2 x$

Logarithms of negative numbers are undefined.

The logarithms of a positive number to the base of the same number are equal to 1.

The bases of an exponential function and its equivalent logarithmic function are equal.

Other properties of logarithmic functions include: The power rule of logarithm states that the logarithm of a number with a rational exponent is equal to the product of the exponent and its logarithm. The quotient rule of logarithms states that the logarithm of the two numbers’ ratio with the same bases is equal to the difference of each logarithm. The product rule of logarithm states the logarithm of the product of two numbers having a common base is equal to the sum of individual logarithms. Properties of logarithmic functions are simply the rules for simplifying logarithms when the inputs are in the form of division, multiplication, or exponents of logarithmic values. To solve an equation with logarithm(s), it is important to know their properties. That means one can undo the other one i.e. The natural log or ln is the inverse of e. To solve the logarithmic functions, it is important to use exponential functions in the given expression. The function f (x) = log b x is read as “log base b of x.” Logarithms are useful in mathematics because they enable us to perform calculations with very large numbers. Then the logarithmic function is given by į(x) = log b x = y, where b is the base, y is the exponent, and x is the argument. We can represent this function in logarithmic form as: An exponential function is of the form f (x) = b y, where b > 0 0 < x and b ≠ 1. Therefore it is useful we take a brief review of exponents.Īn exponent is a form of writing the repeated multiplication of a number by itself.

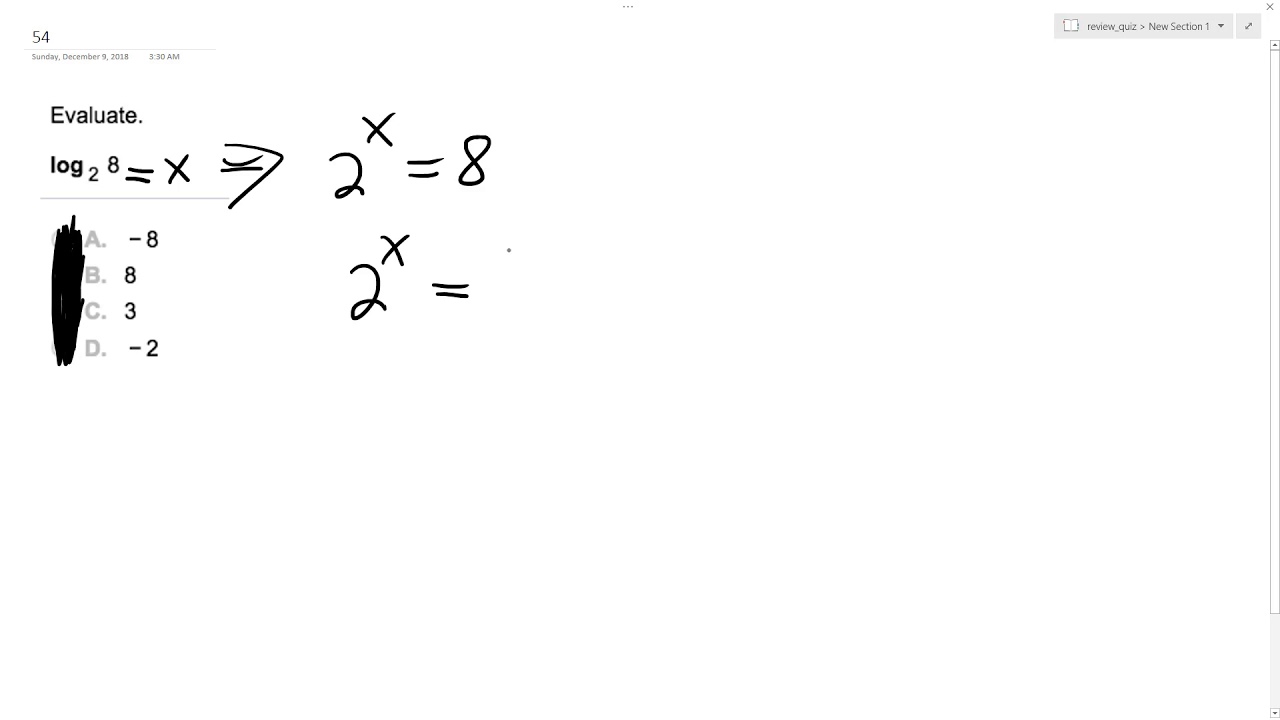
Logarithms and exponents are two topics in mathematics that are closely related. In this article, we will learn how to evaluate and solve logarithmic functions with unknown variables. Solving Logarithmic Functions – Explanation & Examples